
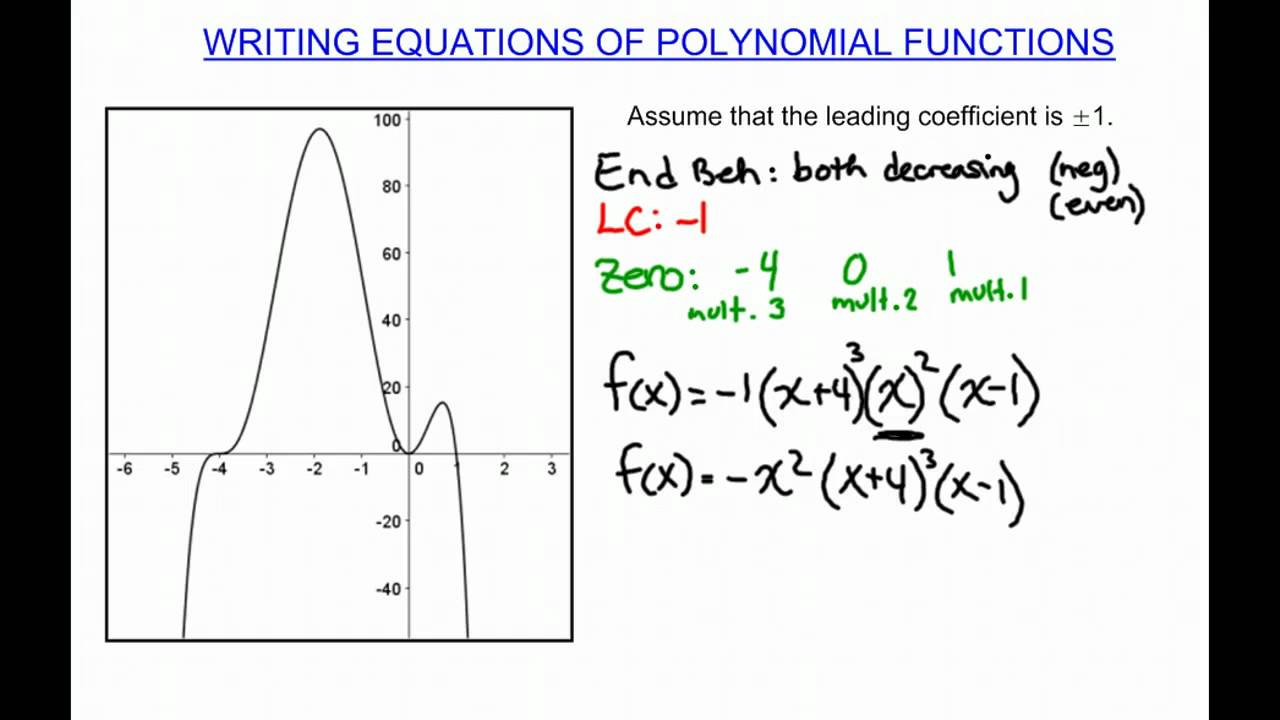
Inflection points: When a graph approaches its inflection point, it flattens as if it were a local extrema, but without changing the course once it passes.These are also known as the maxima and minima of a function. Local extrema: points of the polynomial around which all other values are smaller or larger.At critical points, the derivative of the polynomial equals zero. They describe the graph behavior between the roots: if the values increase or decrease, and until what number they do it. Critical pointsĬritical points are the next step in drawing a polynomial graph. There are formulas for polynomials of third and fourth-degree, but they are long and require tedious work. P(1) = 2*3² - 2*3 - 12 = 18 - 6 - 12 = 0.įinding the root is simple for linear equations (first-degree polynomials) and quadratic equations (second-degree polynomials), but for third and fourth-degree polynomials, it can be more complicated. For example, the polynomial P(x) = 2x² - 2x - 12 has a zero in x = 3 since:

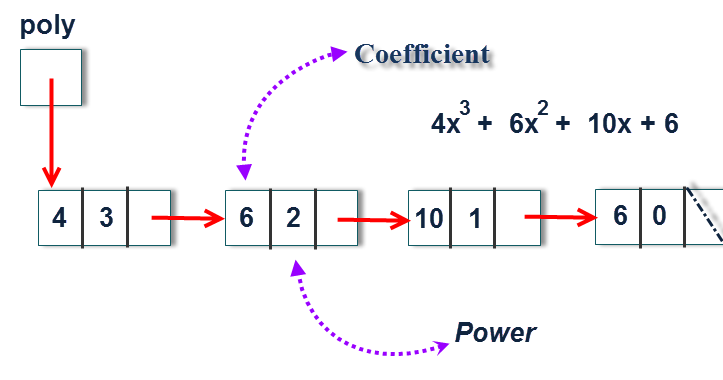
The root or zero of a polynomial is a point in the domain (x-values) where a function equals zero. Generating polynomial graphs manually often requires knowing two features of the polynomial: 1. The calculator will find (with steps shown) the sum, difference, product, and result of the division of two polynomials (quadratic, binomial, trinomial, etc.). Therefore, the letters cannot be, e.g., under roots, in the denominator of a rational expression, or inside a function. The calculator will find (with steps shown) the sum, difference, product, and result of the division of two polynomials (quadratic, binomial, trinomial. Polynomials are algebraic expressions in which variables appear only in non-negative integer powers.


 0 kommentar(er)
0 kommentar(er)
